An Ohm Is A Unit Of ____
Breaking News Today
May 10, 2025 · 7 min read
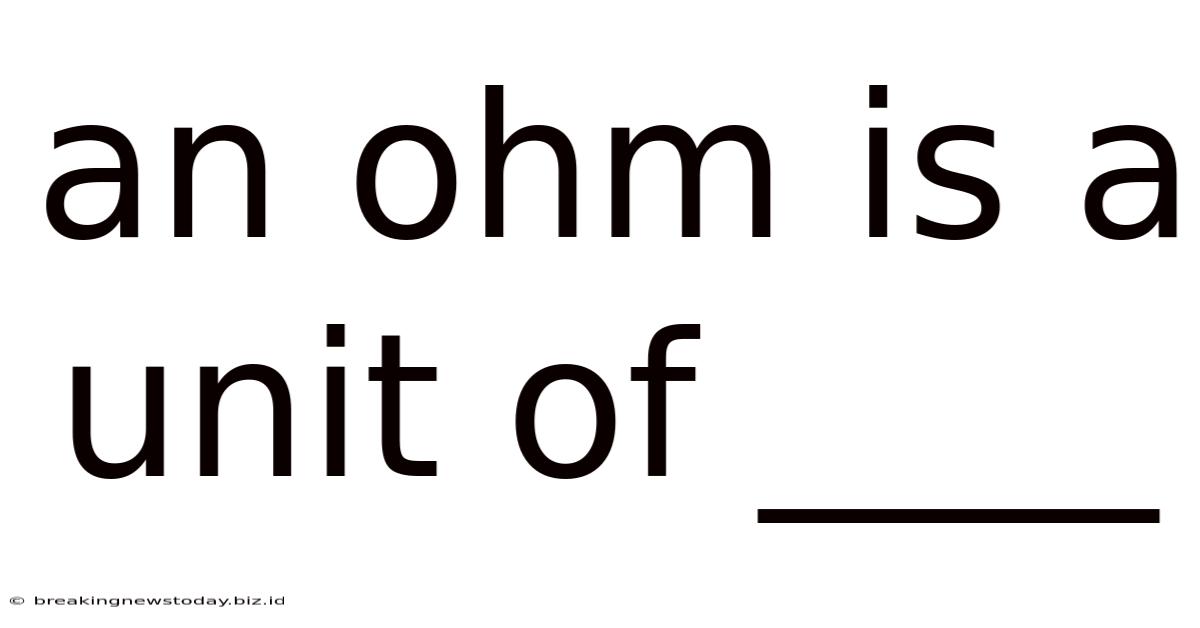
Table of Contents
An Ohm is a Unit of Electrical Resistance: A Deep Dive into Ohm's Law and its Applications
An ohm is a unit of electrical resistance. This seemingly simple statement opens the door to a fascinating world of electricity, electronics, and the fundamental principles governing the flow of current. Understanding what an ohm represents is crucial for anyone working with electrical circuits, from novice hobbyists to seasoned engineers. This comprehensive guide will delve into the intricacies of resistance, exploring its definition, measurement, practical applications, and the vital role it plays in Ohm's Law, the cornerstone of electrical circuit analysis.
Understanding Electrical Resistance
Before we delve into the specifics of the ohm, let's establish a clear understanding of what electrical resistance actually is. Imagine a water pipe. The wider the pipe, the easier it is for water to flow. Similarly, in an electrical circuit, resistance determines how easily electric current flows. A high resistance means the current faces significant opposition, while a low resistance allows current to flow more freely.
Resistance is a property of a material that opposes the flow of electric charge (current). This opposition is caused by the interactions between the moving electrons in the current and the atoms within the material. These interactions convert some of the electrical energy into heat, a phenomenon known as Joule heating or I²R loss.
The Ohm: Defining the Unit of Resistance
The ohm, symbolized by the Greek letter Ω (omega), is the SI unit of electrical resistance. It's defined as the resistance between two points of a conductor when a constant potential difference of one volt, applied to these points, produces a current of one ampere, the conductor not being the seat of any electromotive force.
This definition might seem complex, but it essentially means that if a one-volt battery produces a one-ampere current through a component, that component has a resistance of one ohm. The relationship between voltage (V), current (I), and resistance (R) is elegantly expressed by Ohm's Law, which we'll explore in more detail later.
Factors Affecting Resistance
Several factors influence the resistance of a material:
Material:
Different materials have different intrinsic abilities to conduct electricity. Conductors, such as copper and silver, offer low resistance, while insulators, like rubber and glass, exhibit extremely high resistance. Semiconductors, such as silicon and germanium, fall between these two extremes, and their resistance can be controlled by varying factors like temperature and doping.
Length:
The longer a conductor, the greater its resistance. Imagine a long, thin water pipe – water flow is more restricted than in a short, wide pipe. Similarly, electrons have to travel further in a longer conductor, encountering more obstacles.
Cross-Sectional Area:
The thicker a conductor, the lower its resistance. A larger cross-sectional area provides more pathways for electrons to flow, reducing the overall resistance. Think of multiple water pipes running in parallel—water flow is significantly improved.
Temperature:
Temperature plays a significant role in the resistance of most materials. In general, the resistance of metals increases with increasing temperature. This is because higher temperatures cause increased atomic vibrations, hindering the free flow of electrons. However, some materials, such as semiconductors, exhibit a decrease in resistance with increasing temperature.
Ohm's Law: The Foundation of Electrical Circuits
Ohm's Law is the fundamental principle governing the relationship between voltage, current, and resistance in an electrical circuit. It states:
V = I × R
Where:
- V represents voltage (measured in volts) – the electrical potential difference between two points in a circuit. It's the "push" that drives the current.
- I represents current (measured in amperes) – the rate of flow of electric charge. It's the amount of charge passing a point per unit time.
- R represents resistance (measured in ohms) – the opposition to the flow of current.
Ohm's Law can be rearranged to solve for any of the three variables:
- I = V / R (Current equals voltage divided by resistance)
- R = V / I (Resistance equals voltage divided by current)
This law is incredibly useful for analyzing and designing electrical circuits. Knowing any two of the variables allows you to calculate the third.
Practical Applications of Resistance
Resistance plays a vital role in countless electrical and electronic applications. Here are a few examples:
-
Resistors: These are components specifically designed to introduce a specific amount of resistance into a circuit. They are used to control current flow, divide voltage, and limit power. Resistors come in a wide variety of values, sizes, and power ratings.
-
Heating elements: The resistance of materials like nichrome wire is exploited in heating elements found in appliances such as toasters, electric heaters, and hair dryers. The high resistance generates significant heat through Joule heating.
-
Sensors: Changes in resistance can be used to measure various physical quantities. For example, thermistors change their resistance with temperature, allowing them to be used as temperature sensors. Strain gauges measure changes in resistance due to deformation, providing a means to measure strain and force.
-
Potentiometers: These variable resistors allow for the adjustment of voltage or current in a circuit. They are commonly used in volume controls, dimmer switches, and other applications requiring adjustable resistance.
-
Integrated Circuits (ICs): Resistance is an integral part of integrated circuits. Transistors, which are the building blocks of ICs, rely on precisely controlled resistances to function correctly.
Measuring Resistance
Resistance is typically measured using an instrument called a multimeter. Multimeters are versatile tools capable of measuring voltage, current, and resistance, among other electrical parameters. To measure resistance, you simply select the resistance setting on the multimeter, connect the probes across the component, and read the displayed value in ohms. It is crucial to ensure the circuit is de-energized before attempting any resistance measurements.
Beyond Ohm's Law: Non-Ohmic Devices
While Ohm's Law is a fundamental principle, it doesn't apply to all devices. Non-ohmic devices don't exhibit a linear relationship between voltage and current. Their resistance changes depending on the applied voltage or current. Examples of non-ohmic devices include diodes and transistors. Analyzing these devices requires more advanced techniques beyond the simple application of Ohm's Law.
The Importance of Understanding Resistance in Circuit Design
Understanding resistance is paramount in circuit design. Incorrectly selecting resistor values can lead to:
-
Component damage: If a resistor is rated for a lower power than the actual power dissipated, it may overheat and fail.
-
Circuit malfunction: Incorrect resistance values can disrupt the intended operation of a circuit.
-
Safety hazards: Incorrect resistance values can lead to excessive current flow, posing a fire or shock hazard.
Careful calculation and selection of resistors are essential for creating reliable and safe electrical circuits.
Advanced Concepts: Series and Parallel Resistors
When multiple resistors are connected in a circuit, their combined resistance depends on their arrangement:
-
Series resistors: When resistors are connected in series, their resistances simply add up: R<sub>total</sub> = R<sub>1</sub> + R<sub>2</sub> + R<sub>3</sub> + ...
-
Parallel resistors: When resistors are connected in parallel, the reciprocal of the total resistance is equal to the sum of the reciprocals of the individual resistances: 1/R<sub>total</sub> = 1/R<sub>1</sub> + 1/R<sub>2</sub> + 1/R<sub>3</sub> + ...
Understanding these concepts is crucial for analyzing more complex circuits.
Conclusion: The Ohm's Enduring Significance
The ohm, as a unit of electrical resistance, holds a pivotal position in the world of electronics. Its straightforward definition and the foundational role it plays in Ohm's Law make it indispensable for understanding and manipulating the flow of electric current. From simple circuits to complex integrated systems, a thorough grasp of resistance is fundamental for anyone working with electricity. The ability to accurately calculate and measure resistance is critical not only for ensuring proper circuit function but also for maintaining safety and preventing damage to components. Mastering the concepts discussed here will equip you with the essential knowledge to confidently navigate the fascinating realm of electrical engineering and electronics.
Latest Posts
Latest Posts
-
Which Of The Following Is An Example Of Deductive Reasoning
May 10, 2025
-
What Element Is Shiny And Conducts Heat And Electricity
May 10, 2025
-
What Is Critical When Selecting The Ammunition For A Firearm
May 10, 2025
-
Life Income Joint And Survivor Settlement Option Guarantees
May 10, 2025
-
Which Text Evidence Best Supports The Inference That Jacqueline Overcame
May 10, 2025
Related Post
Thank you for visiting our website which covers about An Ohm Is A Unit Of ____ . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.