The Polynomial 2x3-5x2 4x-10 Is Split Into Two Groups
Breaking News Today
May 09, 2025 · 5 min read
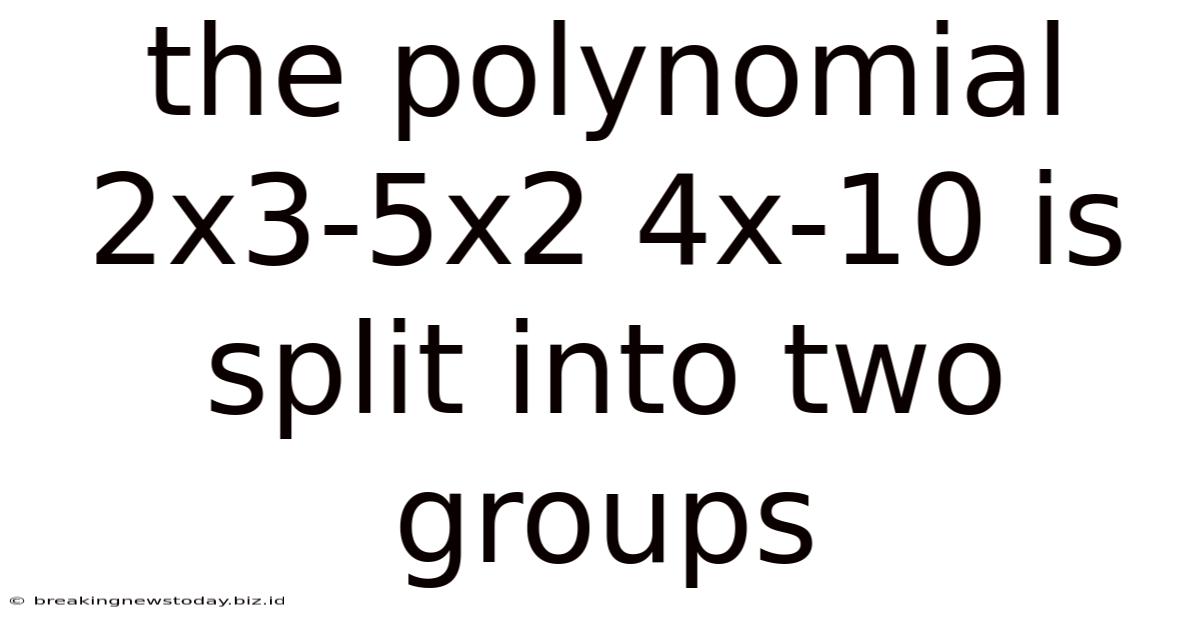
Table of Contents
Exploring the Polynomial 2x³ - 5x² + 4x - 10: A Deep Dive into Grouping and Factorization
The polynomial 2x³ - 5x² + 4x - 10 presents a fascinating case study in algebraic manipulation. While seemingly straightforward, its factorization reveals nuanced techniques and deeper mathematical concepts. This article will explore various methods to analyze this polynomial, focusing particularly on the strategy of grouping terms to facilitate factorization. We will delve into the process, discuss potential pitfalls, and highlight the importance of understanding underlying mathematical principles.
Understanding Polynomial Factorization
Before we delve into the specifics of 2x³ - 5x² + 4x - 10, let's establish a foundation. Polynomial factorization involves expressing a polynomial as a product of simpler polynomials. This process is crucial in various mathematical fields, including calculus, algebra, and even complex analysis. The ability to factor polynomials efficiently simplifies many complex calculations and allows for a deeper understanding of the polynomial's behavior, such as finding its roots (zeros).
Several methods exist for polynomial factorization, including:
- Greatest Common Factor (GCF) method: This involves identifying the largest common factor among all terms and factoring it out.
- Grouping method: This involves grouping terms with common factors to simplify factorization.
- Factoring by difference of squares: This applies to binomials of the form a² - b².
- Factoring by sum/difference of cubes: This method applies to binomials of the form a³ ± b³.
- Quadratic formula: This is used to find the roots of quadratic polynomials and then express them in factored form.
- Synthetic division: This method is used to divide a polynomial by a linear factor.
Applying the Grouping Method to 2x³ - 5x² + 4x - 10
The polynomial 2x³ - 5x² + 4x - 10 is not immediately factorable using the GCF method. However, the grouping method offers a viable pathway. The key is to strategically group the terms in such a way that common factors emerge.
Let's group the terms as follows:
(2x³ - 5x²) + (4x - 10)
Now, let's factor out the GCF from each group:
x²(2x - 5) + 2(2x - 5)
Notice that we now have a common factor of (2x - 5) in both terms. We can factor this out:
(2x - 5)(x² + 2)
Therefore, the factorization of 2x³ - 5x² + 4x - 10 is (2x - 5)(x² + 2).
Analyzing the Factors
We've successfully factored the polynomial using the grouping method. Now let's analyze the resulting factors:
-
(2x - 5): This is a linear factor. Setting it equal to zero allows us to find one of the polynomial's roots: 2x - 5 = 0 => x = 5/2.
-
(x² + 2): This is a quadratic factor. Notice that it cannot be further factored using real numbers because the discriminant (b² - 4ac) is negative (0² - 4 * 1 * 2 = -8). This indicates that the roots of x² + 2 are complex numbers. We can find these complex roots using the quadratic formula:
x = (-b ± √(b² - 4ac)) / 2a
In our case, a = 1, b = 0, and c = 2. Therefore:
x = (± √(-8)) / 2 = ±i√2 (where 'i' is the imaginary unit, √-1)
Thus, the complete set of roots for the polynomial 2x³ - 5x² + 4x - 10 are 5/2, i√2, and -i√2.
Exploring Alternative Grouping Strategies
While the grouping we used above was effective, it's crucial to understand that other grouping strategies might exist, though they may not always lead to a successful factorization using real numbers. For example, we could have grouped the terms differently:
(2x³ + 4x) + (-5x² - 10)
Factoring out the GCF from each group:
2x(x² + 2) - 5(x² + 2)
This again yields (x² + 2)(2x - 5), demonstrating that different grouping strategies can lead to the same factorization. The key is to strategically group terms in a way that reveals common factors.
The Importance of Understanding the Underlying Mathematics
The seemingly simple act of factoring polynomials underscores several fundamental mathematical concepts:
- Distributive Property: The grouping method relies heavily on the distributive property (a(b + c) = ab + ac), allowing us to factor out common terms.
- Fundamental Theorem of Algebra: This theorem guarantees that a polynomial of degree 'n' has exactly 'n' roots (counting multiplicity), which is evident in our analysis of the roots of 2x³ - 5x² + 4x - 10.
- Complex Numbers: The presence of complex roots highlights the importance of expanding our understanding beyond real numbers to encompass the broader field of complex numbers.
Applications of Polynomial Factorization
The ability to factor polynomials is not merely an academic exercise. It has numerous practical applications across various fields:
- Calculus: Factorization is essential for simplifying expressions, finding derivatives and integrals, and analyzing the behavior of functions.
- Engineering: Polynomial factorization is used extensively in solving engineering problems, such as analyzing circuits, designing control systems, and modeling physical phenomena.
- Computer Science: Polynomial factorization plays a vital role in cryptography, algorithm design, and computer graphics.
- Physics: Many physical phenomena are modeled using polynomials, and factorization aids in analyzing and interpreting these models.
Conclusion: Beyond the Numbers
This in-depth analysis of the polynomial 2x³ - 5x² + 4x - 10 demonstrates that even seemingly simple problems can reveal profound mathematical concepts. The grouping method, while appearing straightforward, encapsulates the power of strategic manipulation and the importance of understanding fundamental algebraic principles. Mastering polynomial factorization is a key skill for anyone pursuing advanced studies in mathematics, science, or engineering. The ability to effectively factor polynomials opens doors to a deeper understanding of mathematical structures and their wide-ranging applications in the real world. Beyond the specific techniques, this exploration emphasizes the interconnectedness of mathematical concepts and their relevance to various disciplines.
Latest Posts
Latest Posts
-
Periodic Tune Ups Are Important Because They
May 10, 2025
-
Business Level Strategies Are Concerned Specifically With
May 10, 2025
-
Ban The Ban Sodas A Problem But
May 10, 2025
-
Which Of The Following Is Covered By Health Codes
May 10, 2025
-
Code Range A00 B99 Is Reported In Which Chapter Of Icd 10 Cm
May 10, 2025
Related Post
Thank you for visiting our website which covers about The Polynomial 2x3-5x2 4x-10 Is Split Into Two Groups . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.