Derivatives Of Trig And Inverse Trig Functions
Breaking News Today
Mar 24, 2025 · 6 min read
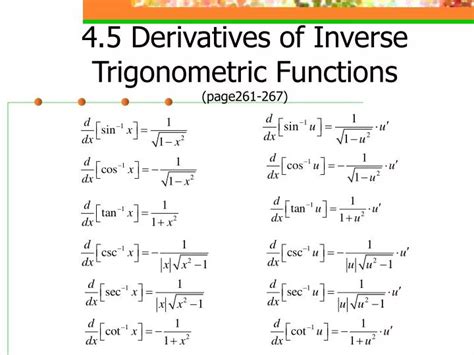
Table of Contents
Derivatives of Trigonometric and Inverse Trigonometric Functions: A Comprehensive Guide
Understanding derivatives is crucial in calculus, and mastering the derivatives of trigonometric and inverse trigonometric functions is essential for tackling more complex problems in calculus, physics, and engineering. This comprehensive guide delves into the core concepts, provides detailed derivations, and offers practical examples to solidify your understanding.
Trigonometric Functions and Their Derivatives
Trigonometric functions describe the relationships between angles and sides in a right-angled triangle. The six primary trigonometric functions are sine (sin), cosine (cos), tangent (tan), cosecant (csc), secant (sec), and cotangent (cot). Their derivatives are fundamental building blocks in calculus.
1. The Derivative of Sine (sin x)
The derivative of sin x is cos x. This can be proven using the limit definition of a derivative and trigonometric identities.
Derivation (using the limit definition):
The limit definition of a derivative is:
f'(x) = lim (h→0) [(f(x + h) - f(x)) / h]
Applying this to f(x) = sin x:
f'(x) = lim (h→0) [(sin(x + h) - sin(x)) / h]
Using the trigonometric identity sin(A + B) = sin A cos B + cos A sin B:
f'(x) = lim (h→0) [(sin x cos h + cos x sin h - sin x) / h]
Rearranging and separating the limit:
f'(x) = lim (h→0) [(sin x (cos h - 1) / h) + (cos x (sin h / h))]
Since lim (h→0) (sin h / h) = 1 and lim (h→0) (cos h - 1) / h = 0, we get:
f'(x) = cos x
2. The Derivative of Cosine (cos x)
The derivative of cos x is -sin x. The derivation follows a similar process to that of sin x, utilizing the limit definition and trigonometric identities.
Derivation (using the limit definition):
f'(x) = lim (h→0) [(cos(x + h) - cos(x)) / h]
Using the trigonometric identity cos(A + B) = cos A cos B - sin A sin B:
f'(x) = lim (h→0) [(cos x cos h - sin x sin h - cos x) / h]
Rearranging and separating the limit:
f'(x) = lim (h→0) [(cos x (cos h - 1) / h) - (sin x (sin h / h))]
Considering the limits mentioned previously, we obtain:
f'(x) = -sin x
3. The Derivative of Tangent (tan x)
The derivative of tan x is sec²x. This can be derived using the quotient rule, since tan x = sin x / cos x.
Derivation (using the quotient rule):
The quotient rule states: (u/v)' = (u'v - uv') / v²
Let u = sin x and v = cos x. Then u' = cos x and v' = -sin x.
Applying the quotient rule:
(tan x)' = [(cos x)(cos x) - (sin x)(-sin x)] / (cos x)²
= (cos²x + sin²x) / cos²x
Using the Pythagorean identity cos²x + sin²x = 1:
(tan x)' = 1 / cos²x = sec²x
4. Derivatives of Other Trigonometric Functions
The derivatives of the remaining trigonometric functions (csc x, sec x, cot x) can be derived using the quotient rule and the derivatives of sin x, cos x, and tan x.
- (csc x)' = -csc x cot x
- (sec x)' = sec x tan x
- (cot x)' = -csc²x
Inverse Trigonometric Functions and Their Derivatives
Inverse trigonometric functions (also called arcus functions or cyclometric functions) are the inverse functions of trigonometric functions. They return the angle whose trigonometric ratio is a given value. The principal values of inverse trigonometric functions are usually restricted to specific ranges to ensure a one-to-one relationship.
1. The Derivative of arcsin x (sin⁻¹x)
The derivative of arcsin x is 1 / √(1 - x²). This can be derived using implicit differentiation.
Derivation (using implicit differentiation):
Let y = arcsin x. Then sin y = x.
Differentiating both sides with respect to x:
cos y (dy/dx) = 1
Solving for dy/dx:
dy/dx = 1 / cos y
Since sin²y + cos²y = 1, we have cos y = √(1 - sin²y). Substituting sin y = x:
dy/dx = 1 / √(1 - x²)
2. The Derivative of arccos x (cos⁻¹x)
The derivative of arccos x is -1 / √(1 - x²). The derivation is similar to that of arcsin x.
Derivation (using implicit differentiation):
Let y = arccos x. Then cos y = x.
Differentiating both sides with respect to x:
-sin y (dy/dx) = 1
Solving for dy/dx:
dy/dx = -1 / sin y
Since sin y = √(1 - cos²y) and cos y = x:
dy/dx = -1 / √(1 - x²)
3. The Derivative of arctan x (tan⁻¹x)
The derivative of arctan x is 1 / (1 + x²). This can also be derived using implicit differentiation.
Derivation (using implicit differentiation):
Let y = arctan x. Then tan y = x.
Differentiating both sides with respect to x:
sec²y (dy/dx) = 1
Solving for dy/dx:
dy/dx = 1 / sec²y
Since sec²y = 1 + tan²y and tan y = x:
dy/dx = 1 / (1 + x²)
4. Derivatives of Other Inverse Trigonometric Functions
The derivatives of the remaining inverse trigonometric functions (arccsc x, arcsec x, arccot x) can be derived similarly, employing implicit differentiation.
- (arccsc x)' = -1 / (|x|√(x² - 1))
- (arcsec x)' = 1 / (|x|√(x² - 1))
- (arccot x)' = -1 / (1 + x²)
Applications and Examples
Derivatives of trigonometric and inverse trigonometric functions are vital in numerous applications:
- Physics: Calculating velocities and accelerations in oscillatory motion (e.g., simple harmonic motion).
- Engineering: Analyzing circuits with sinusoidal signals, designing mechanical systems involving rotating parts.
- Calculus: Solving optimization problems, finding areas and volumes using integration.
Example 1: Finding the slope of a tangent line.
Find the slope of the tangent line to the curve y = sin x at x = π/4.
The slope is given by the derivative: dy/dx = cos x.
At x = π/4, the slope is cos(π/4) = √2/2.
Example 2: Related rates problem.
A ladder 10 meters long rests against a vertical wall. If the bottom of the ladder slides away from the wall at a rate of 2 m/s, how fast is the top of the ladder sliding down the wall when the bottom of the ladder is 6 meters from the wall?
This problem requires using implicit differentiation and trigonometric functions.
Example 3: Optimization problem.
Find the maximum area of a rectangle inscribed in a circle of radius r. This problem uses trigonometric functions to express the area and then employs calculus to find the maximum.
Conclusion
Mastering the derivatives of trigonometric and inverse trigonometric functions is crucial for success in calculus and its many applications. By understanding the derivations and practicing with examples, you can build a strong foundation for tackling more advanced mathematical concepts and real-world problems. Remember to utilize the fundamental trigonometric identities and the appropriate differentiation rules (limit definition, quotient rule, implicit differentiation) to accurately derive these essential derivatives. Consistent practice and problem-solving will further enhance your proficiency.
Latest Posts
Latest Posts
-
When Nucleotides Polymerize To Form A Nucleic Acid
Mar 29, 2025
-
The Average Adult Eats About 4 000 Calories A Day
Mar 29, 2025
-
When Vehicle Wheels Are About To Lock The Abs
Mar 29, 2025
-
The Par Value Per Share Of Common Stock Represents
Mar 29, 2025
-
Earth Science The Physical Setting Answer Key
Mar 29, 2025
Related Post
Thank you for visiting our website which covers about Derivatives Of Trig And Inverse Trig Functions . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
