The Natural Frequency Of An Object Depends On Its
Breaking News Today
Mar 29, 2025 · 7 min read
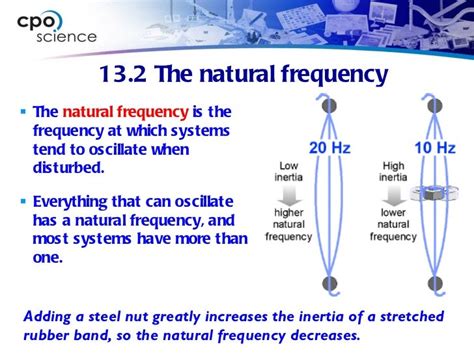
Table of Contents
The Natural Frequency of an Object Depends On: A Deep Dive into Vibration
The world around us is constantly vibrating. From the subtle hum of a power line to the resonant clang of a struck bell, vibrations are ubiquitous. Understanding the factors that govern these vibrations, particularly the concept of natural frequency, is crucial in fields ranging from engineering and physics to music and seismology. This article will explore the multifaceted nature of natural frequency, delving into the key properties of an object that determine its inherent vibrational tendencies.
What is Natural Frequency?
Every object possesses a natural frequency, or more accurately, a set of natural frequencies. This represents the frequency at which the object will vibrate most readily when disturbed. Think of pushing a child on a swing – you need to push at the right rhythm (frequency) to achieve maximum amplitude. Pushing at any other frequency will be less effective. This "right rhythm" is the swing's natural frequency.
In simpler terms: Natural frequency is the inherent tendency of an object to oscillate at a specific rate when energy is introduced, whether it's a brief tap, sustained force, or even a passing sound wave. When an object is subjected to a force at or near its natural frequency, a phenomenon called resonance occurs, leading to dramatically amplified vibrations.
Factors Influencing Natural Frequency
Several key factors influence the natural frequency of an object. These factors are interconnected and often work in concert to determine the object's vibrational behavior.
1. Material Properties: Stiffness and Density
The material an object is made from plays a significant role in its natural frequency. Two crucial material properties are:
-
Stiffness (Young's Modulus): A stiffer material resists deformation more strongly. A stiffer object will have a higher natural frequency. Imagine a guitar string: a thicker, stiffer string will vibrate at a higher pitch (frequency) than a thinner, more flexible string. This is because the stiffer string resists bending more effectively, leading to faster oscillations.
-
Density: A denser material possesses more mass per unit volume. A denser object will have a lower natural frequency. Consider two identical bars, one made of aluminum and the other of steel. Steel, being denser, will have a lower natural frequency than the aluminum bar, even if both have the same stiffness. This is because the greater mass of the steel bar resists acceleration more effectively.
The relationship between stiffness and density often determines the speed of wave propagation within the material, which in turn heavily influences the natural frequency.
2. Geometry and Shape: Size and Dimensions
The physical dimensions and shape of an object significantly impact its natural frequency. For simple systems like strings, rods, or beams, we can use straightforward mathematical formulas to calculate the natural frequencies. However, for more complex shapes, numerical methods such as Finite Element Analysis (FEA) are often necessary.
-
Length: For a vibrating string or rod, increasing the length lowers the natural frequency. Think again of a guitar string: a longer string vibrates at a lower frequency (lower pitch). This is because the longer string has more mass to accelerate and a longer distance to travel during each oscillation.
-
Mass Distribution: The way mass is distributed within an object affects its natural frequency. A uniform mass distribution typically results in simpler vibrational modes. Non-uniform mass distribution, however, can lead to more complex vibrational patterns and multiple natural frequencies.
-
Shape and Cross-section: The shape and cross-sectional area of an object also play a crucial role. A solid cylindrical rod will have different natural frequencies compared to a hollow cylindrical rod of the same length and material. The object's geometry dictates how it responds to forces and the resulting vibrational modes.
3. Boundary Conditions: How the Object is Supported
How an object is supported or constrained influences its natural frequencies. Boundary conditions define the constraints on the object's movement at its edges or supports.
-
Fixed-Fixed: An object fixed at both ends (like a string clamped at both ends) will have higher natural frequencies compared to an object with other boundary conditions.
-
Fixed-Free: An object fixed at one end and free at the other (like a cantilever beam) will have lower natural frequencies.
-
Free-Free: An object free at both ends will have even lower natural frequencies.
Different boundary conditions affect the wavelengths of the vibrational modes and subsequently the natural frequencies.
4. External Factors: Loading and Environmental Conditions
While less fundamental than the material properties and geometry, external factors can still subtly influence the natural frequency.
-
Applied Loads: Adding mass to an object or applying external forces will alter its natural frequency. A loaded structure will generally have lower natural frequencies than an unloaded structure.
-
Temperature: Temperature changes can affect material properties such as stiffness and density, thereby altering the natural frequency. Materials generally become less stiff at higher temperatures.
-
Damping: Damping refers to energy dissipation mechanisms that reduce the amplitude of vibrations over time. Damping can arise from internal friction within the material, air resistance, or other energy loss mechanisms. While damping doesn't directly change the natural frequency, it significantly impacts the decay rate of vibrations. Highly damped systems will quickly lose energy and cease to vibrate, while lightly damped systems will vibrate for longer periods.
Calculating Natural Frequency: Simple Systems
For simple systems, such as a stretched string or a simple pendulum, we can use relatively straightforward formulas to calculate the natural frequency (f). These formulas often involve the object's physical properties and relevant constants.
String's Natural Frequency:
The fundamental frequency (first harmonic) of a stretched string is given by:
f = (1/2L)√(T/μ)
Where:
- f = fundamental frequency
- L = length of the string
- T = tension in the string
- μ = linear mass density (mass per unit length)
Simple Pendulum's Natural Frequency:
The natural frequency of a simple pendulum is given by:
f = (1/2π)√(g/L)
Where:
- f = natural frequency
- g = acceleration due to gravity
- L = length of the pendulum
These are simplified models. Real-world systems are often far more complex and require more sophisticated analytical or numerical methods.
The Significance of Natural Frequency
Understanding natural frequency is critical in numerous applications:
-
Structural Engineering: Engineers must consider the natural frequencies of structures like buildings and bridges to ensure they don't resonate with external forces like wind or earthquakes, potentially leading to catastrophic failure.
-
Mechanical Engineering: Designers of machines and engines need to understand natural frequencies to avoid resonance that could cause excessive vibrations, noise, and component failure. Balancing rotating parts is a crucial aspect of this.
-
Acoustics: Natural frequencies are fundamental to the design of musical instruments, concert halls, and noise control systems. Understanding resonance is essential for optimizing sound production and minimizing unwanted noise.
-
Seismology: The study of earthquakes involves understanding the natural frequencies of the Earth's layers and structures. This knowledge helps in predicting earthquake behavior and mitigating their impact.
-
Medical Imaging: Techniques like ultrasound and MRI rely on understanding how different tissues and organs have different natural frequencies to create images of the body's internal structures.
Resonance and its Implications
Resonance, the phenomenon where an object is subjected to a force at or near its natural frequency, leads to significantly amplified vibrations. While resonance can be harnessed beneficially in musical instruments and other applications, it can also be destructive. The Tacoma Narrows Bridge collapse serves as a stark reminder of the devastating consequences of resonance when a structure's natural frequency is excited by external forces.
Conclusion
The natural frequency of an object is a complex interplay of its material properties, geometry, boundary conditions, and external factors. Understanding these factors is crucial across numerous engineering, scientific, and artistic disciplines. By carefully considering the natural frequency of objects, we can design safer, more efficient structures, instruments, and systems, while also harnessing the power of resonance for constructive purposes. Further research continues to deepen our understanding of vibration and its myriad implications. Advanced techniques like Finite Element Analysis provide increasingly precise tools for predicting and controlling the vibrational behavior of complex systems.
Latest Posts
Latest Posts
-
Functions Of The Liver Include Quizlet Emt
Mar 31, 2025
-
An Application Programming Interface Api Is Quizlet
Mar 31, 2025
-
Dental Disease Dates Back To The Quizlet
Mar 31, 2025
-
Basic Functions Of The Liver Include Quizlet
Mar 31, 2025
-
A Resident On Transmission Based Precautions Must Be Quizlet
Mar 31, 2025
Related Post
Thank you for visiting our website which covers about The Natural Frequency Of An Object Depends On Its . We hope the information provided has been useful to you. Feel free to contact us if you have any questions or need further assistance. See you next time and don't miss to bookmark.
